Who rules the subatomic world?

June 5, 2018 by Nuno Rosa Agostinho
The building blocks of matter
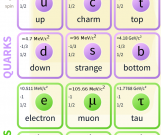
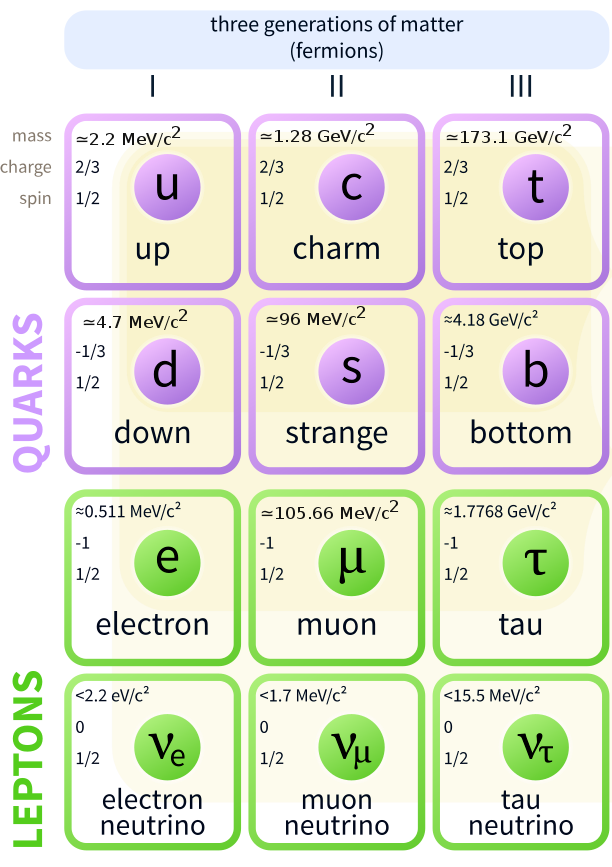
We are made of atoms, the basic units of matter. Everything is made out of them, from the simplest rock to the farthest supernova in our Universe. But even being the basic unit of matter, atoms, are hiding something truly astonishing - leptons and quarks, the true building blocks of matter.
Much like our society is organized, these particles came in “families, hierarchies and colours”.
The quarks are made of three families (or flavours) and each family has always two members, no more no less: the first family of quarks is constituted by the up quark the down quark; the second family is also represented by two quarks, the charm quark and the strange quark; a third family with the top quark and his fellow bottom quark.
What about the hierarchy? The three families are hierarchical in terms of the mass, being the third family the heaviest one and the first family the lightest one. As an example, if one takes the ratio between the mass of top quark and the up, one will get that the top quark is 75308.7 times heavier than the up quark. As a result, the ordinary matter is constituted essentially by the quarks of the first generation, while the heavy quarks decay with a very short half-life and are only observed in high energy environments, like particle accelerators.
Finally, we are missing also one important property of quarks that distinguishes them from other particles, the coulour. Each quark can have three colours, red, blue and green. Not real colours, it is just an analogy, since quarks are too small to have a perceptual characteristic like colour.
There is an interesting property about quarks and that is, perhaps, one of the main reasons to use this analogy with ordinary colours. Quarks can’t stay apart, and they like to combine such that the result is an colourless particle, much inspired in the human vision, where red light plus green light plus blue light appears as "colorless" white light. In practice, they like to hide the colour from the macroscopic world. When they combine, any colour is favored, which means that ordinary matter is colourless.
If we consider the proton, we see that it is made of two up quarks, one is red and the other is blue, and one green down quark ( remember that proton is one of the most stable particles, being made only by light quarks).
The leptons follow some of the same principles of the quarks society, having the same number of families with two elements, a particle and an antiparticle (particle with the same mass but opposite electrical charge).
The first lepton family is formed by the electron, on the second family we have the muon and finally the third family is composed by the tau lepton.
Regarding the hierarchy of masses, the families of leptons have the same behavior of the quarks. Although they don’t share the same colour properties of quarks, being directly colourless.
Furthermore, it is worthy to mention other characteristic of the elementary particles named spin. Spin in particle physics is a pure formulation of Quantum Mechanics and it is an instrinsic porperty of a particle, like the charge, at least at this level we should do not try to make any analogy with what whappens in the macroscopic world and rotating objects.
All these particles that have been described in the above, fermions, have spin ½ (half integer) cannot occupy the same place at the same time. In a quantum description, they cannot have the same quantum numbers.
If you have been mindful to the orgaziation of these lego pieces of the Standard Model, you noticed a peculiar fact. Quark families have two member, but what about leptons? Just only one member?! Not really...
The elusive brothers
Until the moment, we only described the lepton family as being composed only by charged elementary particles, however the story is far more intricate. The truth is that there are other particles that do not have charge and interact weekly with the matter, which make them difficult to detect.
These particles were postulated by Enrico Fermi, in 1930, in order explain the conservation of energy momentum in the beta decay, where the neutron decays to a proton, to a electron and to an weekly interacting neutral particle, a neutrino (to be more precise, it is an antineutrino, the neutrino antiparticle).
Like the other leptons, neutrinos are fermions associated in three families. We have the electron neutrino, the muon neutrino and the tau neutrino, and they have a peculiar ability of changing flavour. How? Astonishingly, if we produce a neutrino of muon type, after a while it became a neutrino of electron type. This periodic phenomea is called neutrino oscillations.
Fundamental interactions
Proceeding with the analogy of our civilization, particles need to communicate with each other.
Different languages arise when we take into account each type of particle.
Let’s first consider the charged ones. Charged particles can communicate among themselves by interchanging a particle called photon – the carrier of the electromagnetic force. Whenever an electron is orbiting a nucleus or there is some kind of repulsion between two electrons, the exchange of (virtual) photons is responsible for it. Moreover the photon is massless, uncharged and have unlimited range, which makes the electromagnetic force the one with longest range (indeed infinite range).
As mentioned above, the family of quarks also like to stick together and sometimes be apart of the world, speaking a language that the other elementary particles don’t know. Thus, quarks can communicate trough the exchange of massless particles called gluons, the carriers of the strong nuclear force. Contrary to the photons, gluons cannot reach much beyond the nucleus, which makes the strong force a short range one.
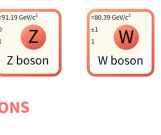
Families of particles also have a common language to all of them and we shall call it weak interaction. In the weak interaction, particles exchange three new particles, the neutral Z particle and the charged W+, W- particles. A very common example is the decay of a neutron to a proton, that involves the “exchange of a W- particle”.
We know that there four fundamental forces, thus one is missing from the picture. We are talking about gravity and it does not enter in the subatomic world, yet. Physicist have faith that someday a theory of everything, that glues gravity to the other three fundamental forces, “merging” the macroscopic world to the microscopic one, will be proposed and experimentally confirmed.
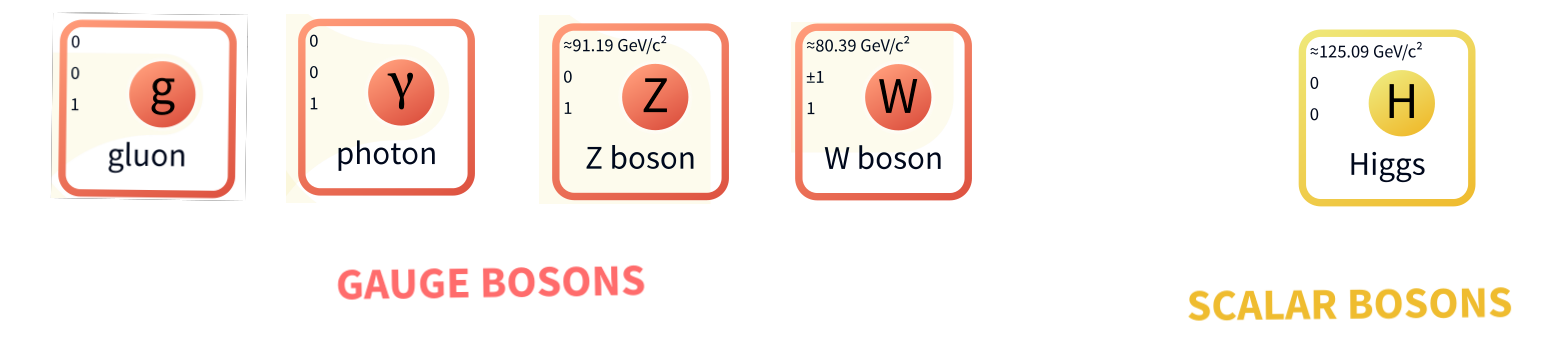
With the description of the fundamental forces, the particle content enlarged with new particles, and we can form a new group, the bosons. The charged particles W+ and W-, the neutral Z, the photon and the gluon belong to the bosons. These groups of particles obey to the Bose-Einstein statistical rules.
Contrary to the fermions, bosons do not have any particular problem on occupying the same place at the same time, which in terms of Quantum Mechanics means that two or more bosons may be described by the same quantum numbers, and have an integer number for the spin.
One particle is missing...and believe that the role of this particle is not to be despised. Do you know why quarks have mass but the gluons don't? Or why do the W and Z bosons have mass but the photon doesn't? Maybe there is a different interaction, that only some particles feel. A new interaction is synonymous of a new particle and this particle does not like to hang out with every one that is resting, just picks some of them to go to a good restaurant and pays a really nice meal until they start to gain some weight.
Higgs boson is its name, a scalar particle with spin 0, and was proposed by François Englert, Robert Brout and Peter Higgs. It was discovered in 2012 at the LHC. The rest of the story should be completed after discussing the concept of symmetries.
What glues everything? Symmetries
Symmetries always were important in our civilization. Since the ancient Greece, symmetries were associated to description of objects, to the description of the nature. Kepler imposed the notions of symmetries to explain the motion of the planets.
Symmetry principles play an important role with respect to the laws of nature as we can see from Newtons theory, Galileo invariance and Maxwell equations, but not in an explicit way, in the sense that the dynamical equations were already given after identifying the physical problem, and afterwards was just a question of study the symmetries of the problem.
But nowadays we mainly follow a different approach: start with specific symmetries and search for dynamical equations with such properties. So, if symmetries summarize the regularities of the laws that are independent of dynamics – invariance – than we can find a structure and coherence for the laws.
Why invariance is important? For example the invariance of laws under the space-time translations is what makes us capable of repeating experiments at different places and different times. Symmetries dictate the form of laws of nature!
If we took the concept of symmetry from the macroscopic world to the quantum level, something magic happens! Symmetries and invariance are connected with conservation laws.
For example, the invariance under space translations is associated with the conservation of the momentum. This is well know as a corollary of Noether’s theorem.
Moreover, a Chinese physicist, Nobel prize winner, T.D. Lee went beyond Noether’s theorem and introduced the concept of non-observables:”The root of all symmetry principles lies in the assumption that it is impossible to observe certain basic quantities; these will be called ‘non-observables’”. So, right now you have to deal with the reality. To a symmetry or invariance principle, there is not only associated a conservation law but a also a non-observable.
Continuing to the same example, the invariance under space translations is now associated with the conservation of the momentum and the lack of knowledge of the absolute position in space, our non-observable.
Symmetry |
Conservation Laws |
Non-observable |
| Space translation | Momentum | Absolute position in space |
| Time translation | Energy | Absolute time |
| Rotation | Angular momentum | Absolutes pace direction |
| Space reflection | Parity | Absolute handedness |
| Phase transformation | Charge | Absolute charge sign |
| Permutation | Bose-Einstein statistics and Fermi-Dirac statistics | Diference between identical particles |
| Lorentz transformations | Generators of Lorentz group | Absolute velocity |
So, what happens if somehow we can observe one of these non-observables? Symmetry breaking is the answer, and we are getting close to the role of the Higgs boson in the Standard Model.
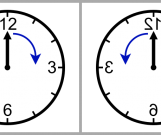
The first evidence of this phenomena was in the violation of the parity in the nuclear beta decay, by the physicst Wu, at 1957. Parity can be understood using the following figure.
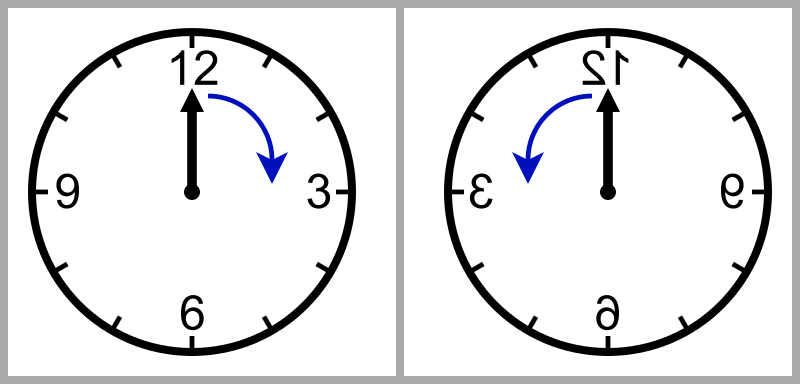
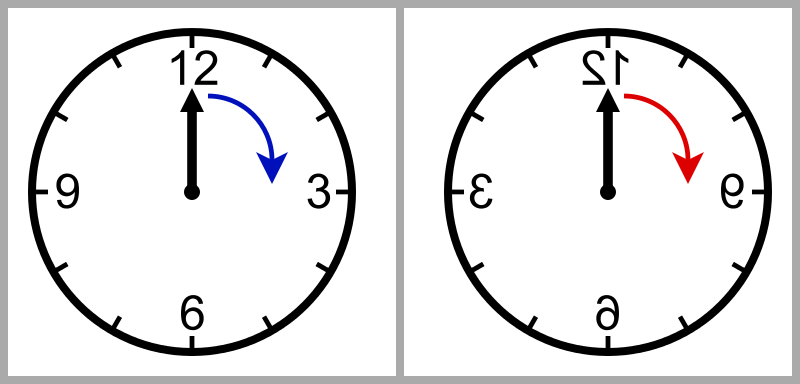
The left figure shows parity symmetry: A clock works like its mirrored image. On the other hand, the right figure shows parity asymmetry, where the clock built does not work as its mirrored image.
It seems that the parity is not an symmetry of weak interactions. During the beta emission, most of the particles had a preferred direction of decay relative to the nuclear spin.
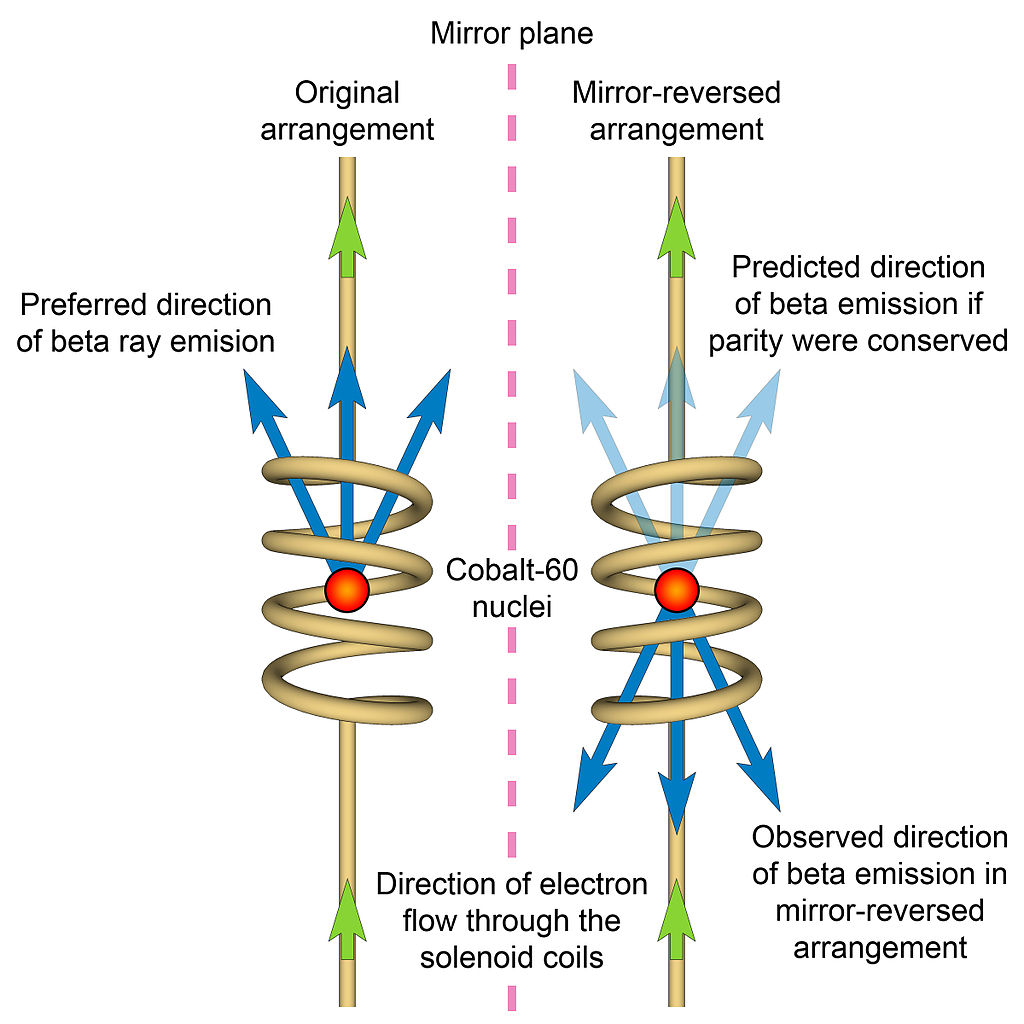
Now, we know that symmetries can be broken and there is two types of symmetry breaking. The one interesting for particle physics is the spontaneous symmetry breaking. In this case, the symmetries are not completely broken but simply hidden in the low energy state, like the cold ferromagnet hides the spherical symmetry inherent in electromagnetism.
Are we have everything to build the Standard Model? Almost. We need just to understand how the particles got massive through the Higgs Boson.
The Standard Model of particle physics is described by having the following combination of symmetries, SU(3)xSU(2)xU(1). Each one tells to the particles how to behave in the society: SU(3) is associated to the strong interaction, SU(2) to the weak interaction and U(1) to the electromagentic interaction.
There are some complicated concepts behind these symmetries, and we would need to know some group theory, but let’s keep the things simple. Each symmetry has an associated conserved quantity and generators. The space is filled with a Higgs field such that its potential breaks the SU(2)xU(1) to the U(1), and since the SU(2) has three generators, there will be three massless Goldstone bosons, by the Nambu-Goldstone theorem.
The hungry W+- and Z bosons will eat the Goldstone bosons, acquiring the so desired mass. Since the S(3) and U(1) remains unbroken, the photon and the gluons remain massless, as well as the color and electric charge are conserved. The remaining fermions swimming through the background sea of Higgs field, interact with it and acquire mass. The more massive the particle, the more it interacted with this field. Finally brace yourself for the funniest part. The Higgs boson interacts with the Higgs field, so it gives mass to itself.
Now we have the complete picture of the Standard Model as simple as a bunch of elementary particles that like to go around and communicate, ruled by a group of symmetries.
Unfortunatelly the picture is not complete..
After this description of what is the Standard Model, we could quote Lord Kelvin “There is nothing new to be discovered in physics now; all that remains is more and more precise measurement”, however that’s far from being truth, even to a successful model as the Standard Model.
One of the main problems is the explanation the mass of the lightest neutrinos. The Standard Model wrongly predicted massless neutrinos and they are ruled out after the detection of neutrinos oscillations.
Why three is so special regarding the flavour puzzle? There is no answer for that, we do not have a clue why there are exactly three families and why the third is the heaviest. Some symmetry could be acting behind it.
The Standard Model is clearly not the end of the story...
-
Did you know that the european research center, CERN, where is located one of the important colliders, LHC, to test the Standard Model and search for possible extension, was the birthplace of the World Wide Web?
This was an answer to the needs of storing a big amount of data to analyse from the runs.